JEE Main Latest Updates
30 Apr, 2025 hiii new single wahtsnew
JEE Main Mathematics Syllabus PDF consists of chapters such as 3-D Geometry, Application of Derivatives, Definite Integration, Sequences & Series, Differentiation, Matrices, etc. In JEE Main Question Paper, a total of 30 questions are asked from the mathematics syllabus of which candidates are required to answer 25 questions. Check JEE Main Mathematics Exam Pattern
The mathematics section in JEE Main Question Paper is considered to be easy to moderate. JEE syllabus for mathematics is a combination of relatively easy and difficult topics. As per a majority of test takers 25% of the questions are easy, 25% difficult and the rest 50% are observed to be of a medium difficulty level. Check JEE Main Mathematics Question Paper
JEE Main Mathematics Syllabus PDF Download
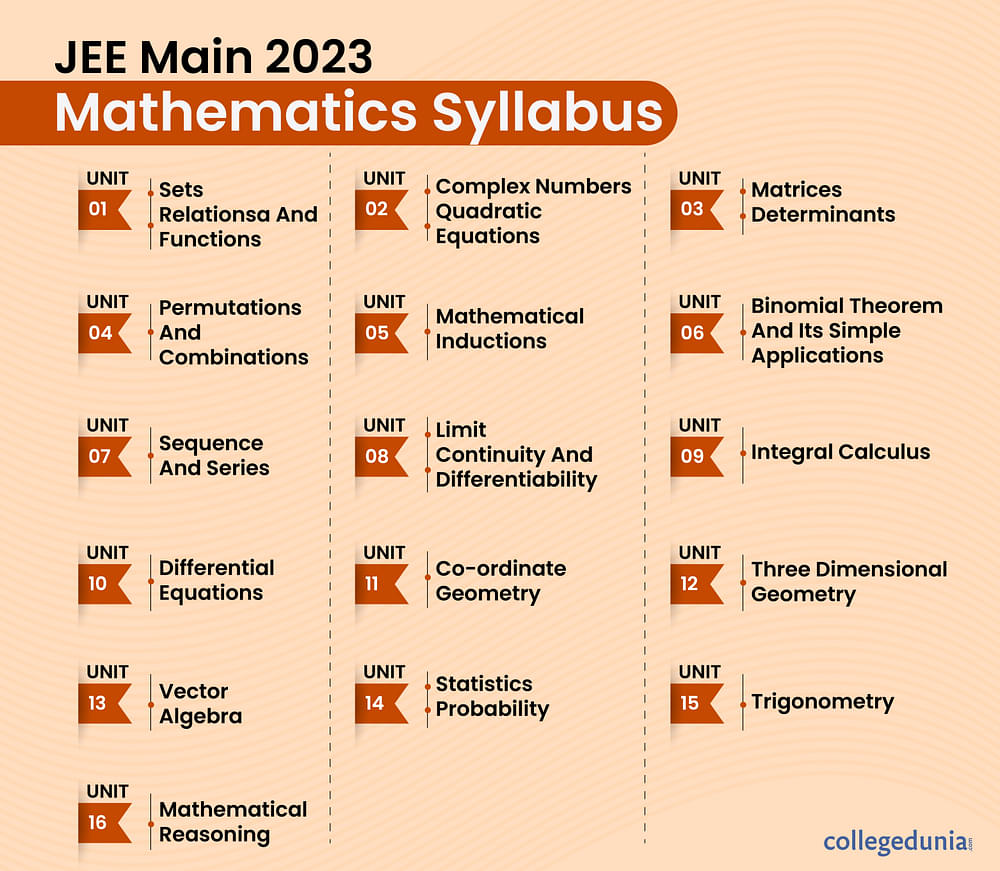
| JEE Main Mathematics Syllabus PDF- Download Here |
JEE Main Mathematics Syllabus: Topics and Sub-Topics by NTA
JEE Main Mathematics syllabus PDF is spread over 18 units covering topics from Class 11th and 12th NCERT.
| Topics | Sub-Topics |
|---|---|
| UNIT 1: SETS, RELATIONS, AND FUNCTIONS | Sets and their representation: Union, intersection and complement of sets and their algebraic properties; Power set; Relation, Type of relations, equivalence relations, functions; one-one, into and onto functions, the composition of functions. |
| UNIT 2: COMPLEX NUMBERS AND QUADRATIC EQUATIONS | Complex numbers as ordered pairs of reals, Representation of complex numbers in the form a + ib and their representation in a plane, Argand diagram, algebra of complex number, modulus and argument (or amplitude) of a complex number, square root of a complex number, triangle inequality, Quadratic equations in real and complex number system and their solutions Relations between roots and co-efficient, nature of roots, the formation of quadratic equations with given roots. |
| UNIT3: MATRICES AND DETERMINANTS | Matrices, algebra of matrices, type of matrices, determinants, and matrices of order two and three, properties of determinants, evaluation of determinants, area of triangles using determinants, Adjoint, and evaluation of inverse of a square matrix using determinants and elementary transformations, Test of consistency and solution of simultaneous linear equations in two or three variables using determinants and matrices. |
| UNIT 4: PERMUTATIONS AND COMBINATIONS | The fundamental principle of counting, permutation as an arrangement and combination as section, Meaning of P (n,r) and C (n,r), simple applications. |
| UNIT 5: MATHEMATICAL INDUCTIONS | Principle of Mathematical Induction and its simple applications. |
| UNIT 6: BINOMIAL THEOREM AND ITS SIMPLE APPLICATIONS | Binomial theorem for a positive integral index, general term and middle term, properties of Binomial coefficients, and simple applications. |
| UNIT 7: SEQUENCE AND SERIES | Arithmetic and Geometric progressions, insertion of arithmetic, geometric means between two given numbers, Relation between A.M and G.M sum up to n terms of special series; Sn, Sn2, Sn3. Arithmetico-Geometric progression. |
| UNIT 8: LIMIT, CONTINUITY, AND DIFFERENTIABILITY | Real–valued functions, algebra of functions, polynomials, rational, trigonometric, logarithmic, and exponential functions, inverse function. Graphs of simple functions. Limits, continuity, and differentiability. Differentiation of the sum, difference, product, and quotient of two functions. Differentiation of trigonometric, inverse trigonometric, logarithmic, exponential, composite and implicit functions; derivatives of order up to two, Rolle’s and Lagrange's Mean value Theorems, Applications of derivatives: Rate of change of quantities, monotonic increasing and decreasing functions, Maxima and minima of functions of one variable, tangents and normal. |
| UNIT 9: INTEGRAL CALCULUS | Integral as an anti-derivative, Fundamental Integrals involving algebraic, trigonometric, exponential, and logarithms functions. Integrations by substitution, by parts, and by partial functions. Integration using trigonometric identities. Integral as limit of a sum. The fundamental theorem of calculus, properties of definite integrals. Evaluation of definite integrals, determining areas of the regions bounded by simple curves in standard form. |
| UNIT 10: DIFFERENTIAL EQUATIONS | Ordinary differential equations, their order, and degree, the formation of differential equations, solution of differential equation by the method of separation of variables, solution of a homogeneous and linear differential equation of the type |
| UNIT 11: CO-ORDINATE GEOMETRY | Cartesian system of rectangular coordinates in a plane, distance formula, sections formula, locus, and its equation, translation of axes, the slope of a line, parallel and perpendicular lines, intercepts of a line on the co-ordinate axis. Straight line Various forms of equations of a line, intersection of lines, angles between two lines, conditions for concurrence of three lines, the distance of a point form a line, equations of internal and external by sectors of angles between two lines co-ordinate of the centroid, orthocentre, and circumcentre of a triangle, equation of the family of lines passing through the point of intersection of two lines. Circle, conic sections A standard form of equations of a circle, the general form of the equation of a circle, its radius and central, equation of a circle when the endpoints of a diameter are given, points of intersection of a line and a circle with the centre at the origin and condition for a line to be tangent to a circle, equation of the tangent, sections of conics, equations of conic sections (parabola, ellipse, and hyperbola) in standard forms, condition for Y = mx +c to be a tangent and point (s) of tangency. |
| UNIT 12: THREE DIMENSIONAL GEOMETRY | Coordinates of a point in space, the distance between two points, section formula, directions ratios, and direction cosines, the angle between two intersecting lines. Skew lines, the shortest distance between them, and its equation. Equations of a line and a plane in different forms, the intersection of a line and a plane, coplanar lines. |
| UNIT 13: VECTOR ALGEBRA | Vectors and scalars, the addition of vectors, components of a vector in two dimensions and three-dimensional space, scalar and vector products, scalar and vector triple product. |
| UNIT 14: STATISTICS AND PROBABILITY | Measures of discretion; calculation of mean, median, mode of grouped and ungrouped data calculation of standard deviation, variance and mean deviation for grouped and ungrouped data. Probability: Probability of an event, addition and multiplication theorems of probability, Baye's theorem, probability distribution of a random variate, Bernoulli trials, and binomial distribution. |
| UNIT 15: TRIGONOMETRY | Trigonometrical identities and equations, trigonometrical functions, inverse trigonometrical functions, and their properties, heights, and distance. |
| UNIT 16: MATHEMATICAL REASONING | Statement logical operations and, or, implies, implied by, if and only if, understanding of tautology, contradiction, converse, and contrapositive. |
Must Read
- How to Effectively Prepare for the Coming JEE Main Session from Home?
- Top 10 Android apps for JEE Main 2023 Preparation
JEE Main Mathematics Syllabus Weightage
As per the previous year trends, here is the topic wise weightage for JEE Main mathematics:
| Chapters | JEE Mathematics Questions | |
|---|---|---|
| June Session (2022) | July Session (2022) | |
| Application of Derivatives | 25 | 16 |
| Area Under Curves | 11 | 8 |
| Basic of Mathematics | 2 | 1 |
| Binomial Theorem | 15 | 11 |
| Circle | 12 | 9 |
| Complex Number | 13 | 13 |
| Continuity & Differentiability | 8 | 7 |
| Definite Integration | 20 | 20 |
| Determinants | 10 | 5 |
| Differential Equations | 19 | 17 |
| Differentiation | 6 | 3 |
| Ellipse | 7 | 5 |
| Functions | 14 | 11 |
| Heights and Distances | 2 | 5 |
| Hyperbola | 8 | 9 |
| Indefinite Integration | 2 | 2 |
| Inverse Trigonometric Functions | 7 | 5 |
| Limits | 13 | 7 |
| Mathematical Reasoning | 13 | 10 |
| Matrices | 17 | 16 |
| Parabola | 12 | 9 |
| Permutation Combination | 13 | 8 |
| Probability | 13 | 13 |
| Properties of Triangles | 1 | 0 |
| Quadratic Equation | 10 | 11 |
| Sequences and Series | 17 | 19 |
| Sets and Relations | 6 | 5 |
| Statistics | 7 | 4 |
| Straight Lines | 9 | 10 |
| Three Dimensional Geometry | 25 | 20 |
| Trigonometric Equations | 5 | 4 |
| Trigonometric Ratios & Identities | 7 | 3 |
| Vector Algebra | 16 | 14 |
JEE Main Mathematics Weightage
| Topics | 2021 | 2020 | 2019 |
|---|---|---|---|
| Three Dimensional geometry | 56 | 19 | 31 |
| Sequences and Series | 38 | 28 | 26 |
| Application of Derivatives | 36 | 31 | 22 |
| Definite Integration | 48 | 15 | 23 |
| Binomial Theorem | 38 | 19 | 21 |
| Differential Equations | 43 | 16 | 15 |
| Determinants | 29 | 21 | 22 |
| Probability | 32 | 17 | 19 |
| Vector Algebra | 35 | 13 | 16 |
| Complex Numbers | 30 | 17 | 14 |
| Quadratic equations | 23 | 16 | 20 |
| Circle | 29 | 8 | 21 |
| Functions | 32 | 11 | 15 |
| Mathematical Reasoning | 25 | 16 | 16 |
| Permutation Combination | 26 | 13 | 14 |
| Matrices | 33 | 10 | 9 |
| Straight Lines | 19 | 12 | 20 |
| Statistics | 21 | 15 | 14 |
| Limits | 24 | 13 | 12 |
| Continuity and Differentiability | 23 | 9 | 13 |
| Area under Curves | 17 | 11 | 14 |
| Parabola | 14 | 11 | 15 |
| Ellipse | 15 | 11 | 9 |
| Indefinite Integration | 11 | 8 | 15 |
| Inverse Trignometric Functions | 19 | 4 | 8 |
| Hyperbola | 10 | 7 | 11 |
| Sets and Relations | 10 | 8 | 8 |
| Trigonometric Equations | 11 | 4 | 8 |
| Differentiation | 5 | 9 | 9 |
| Heights and Distances | 8 | 4 | 7 |
| Trigonometric Ratios and Identities | 7 | 4 | 7 |
| Properties of Triangle | 5 | 0 | 5 |
| Basic of Mathematics | 4 | 0 | 1 |
| Pair of Lines | 2 | 0 | 0 |
| Mathematical Induction | 1 | 0 | 0 |
| Linear Programming | 1 | 0 | 0 |
Maximize Your Score in JEE Main 2023 Mathematics
- Prioritize Calculus and Algebra: Since these two topics contribute a major portion of JEE Main Mathematics Question Paper, candidates must be through with them by practice and any sort of doubt clarification.
- Algebra is one of the easiest and most scoring bits of JEE Main mathematics syllabus. All candidates need to deal with this section is clarity on basics and computational speed. Algebra questions are mostly clubbed with other units and therefore covering algebra will assist in the preparation of the rest.
- Calculus is among the largest sections of modern mathematics, it has two main sections - Differential and Integral Calculus. Questions can be asked in both ways i.e. based on theory and applications.
- Tackling Coordinate Geometry: Considering that most of the questions from this topic are formula based, it can come across as the easiest section for some. However, one must not take this section lightly, a lot of practise is required to avoid silly mistakes in this topic.
- Study all Formulae and Prepare a list: A strong hold on formulae in mathematics is a major advantage in the exam. Candidates must make sure that they have a clarity on all formulas in geometry, trigonometry, probability and of course calculus. During the preparation period, they can keep making a list of formulas for the time of revision.
- Application of concepts: Candidates should revise the applications of geometry and differential equations. This applies for properties of definite integrals and results of algebraic calculations of properties of conics. Thorough revision of these applications should be done before JEE Main exam day.
- Previous year question papers and mock tests: Before you plan your preparation, a look at previous year question papers and mock tests will help your plan move in the right direction.
- Accurate Reference Material: Before you start with your preparation, make sure the reference material you are using is accurate. Also don’t focus on covering all the books, you should have enough material for clarification of concepts and practice.
- Early on Revision: Most often than not candidates wait to cover the entire syllabus and then start revising. However, with a subject like mathematics, to keep your hold on concepts fresh, you must revise at regular intervals. This will also save your time when you sit for complete rounds of revision. So while making a preparation plan candidates must allow sufficient time for revision.
Topic-wise Tips by IITians and Experts for JEE Main Mathematics
We have gathered some valuable inputs by IITians for the preparation of some JEE Main mathematics topics:
Complex Numbers and Quadratic Equations
- The complex numbers and quadratic equations is an important chapter not only just at the school level but any phase of one's technical life. If we talk about JEE Main 2023 syllabus then from the last three to four years one question has been asked from both complex numbers and quadratic equations each.
- Maths is all about practice but there has to be a strategy to prepare for exams. The first thing you need to do is to go through the theory first from a standard book and practice the solved examples to get a hang of the topic.
- When you are comfortable enough with the basic questions, you can check the previous year's question of the complex number and quadratic equation of whichever exam you are aiming for, whether it be school or JEE Main.
- Now comes the practice, pick a question bank book, and keep practicing until you can nail all types of questions that can be formed from these chapters. You can also enroll in all India test series to check your stand against your competitors in case you are preparing for competitive exams.
- If you are preparing for school exams, “I suggest you practice questions at home with a timer. But don't overdo it. If you can solve 10 questions from Complex number and quadratic equations each day, it is enough, as you have other subjects to study too.”
Here are some basic sample questions from Complex Numbers and quadratic equations
- (x + yi) / i = ( 7 + 9i ) , where x and y are real, what is the value of (x + yi)(x - yi)?
- Determine all complex number z that satisfy the equation: z + 3z' = 5 - 6i, where z' is the complex conjugate of z
- How many real roots does the equation have? : x^2 + 3x + 4 = 0
- Evaluate 1/(i^78)
Best books for preparing complex numbers and quadratic equations are:
- NCERT- First complete this for school purpose
- RD Sharma- After NCERT solve all questions of this book whether you are IIT aspirant or not
- Now coming to JEE Main preparation, for this you need some high-level books like ML Khanna, A Dasgupta, Arihant Series, and so on.
- Keep practicing previous years' papers.
Coordinate Geometry
- Coordinate geometry comes in the category of one of those topics where you could score marks quite easily, given the condition enough practice of questions is done.
- This is really an encouraging topic, where you can simply solve a question just by drawing a rough figure.
- Unlike other topics such as calculus or combinatorics where you have to think and do, coordinate geometry is a practical section in which you could actually "see'' what is happening. JEE Main syllabus comprises dealing only with cartesian coordinates, although a little introduction related to polar coordinates is also included.
- A detailed and to the point syllabus for this topic is: Straight lines,pair of straight lines,various forms of straight lines, intersection of lines and angles between two lines, conditions for the concurrence of three lines, distance of a point from line, bisectors;conic sections, conditions for the standard conic equation to be specific conics such as parabola, hyperbola, ellipse, circle, equation of tangents and normals to conic sections, different forms of equations of these conics, different forms of equations of tangents and normals, parametric forms of equations.
- On an average, in JEE Main expected number of questions ranges from 4 to 6 from this topic.
- JEE Main 2019 question paper included a total of 4 questions from coordinate geometry out of the 16 questions which were asked from various topics of class 11th syllabus. (25 % weightage of 11th syllabus and 13.33% overall)
- On an average in JEE Advanced the expected no. of questions from this topic is 6 to 7 ( Both paper 1 & 2 combined) of an easy to moderate difficulty level.
Best books for preparing Coordinate Geometry are:
- SK Goyal has some interesting and advanced level questions.The book starts from a basic level and gradually takes you up to the advanced level. Some corollary to the important theorems are also provided which could be really handy in JEE Advanced. Tons of solved examples are present, which could prove to be really helpful to understand some tricky concepts.
- Some other authors/publications that can be followed are SL Loney, Cengage, Balaji and Disha.
- SK Goyal is recommended apart from solving NCERT questions for boards. Previous year JEE Main and Advanced questions are strongly recommended.
Solve NCERT thoroughly in case you have your board exams. Also solve previous years question papers of your respective board. For JEE Main and JEE Advanced, solve previous years question papers apart from solving SK Goyal or any book which suits you. Proceed to give some tests based on the topic to strengthen and clarify your concepts further. Revision here is key for this topic because of a number of equations, so keep revising.
Some of the frequently asked questions related to the topic are:
Ques. The locus of the point of intersection of the lines xcost+(1-cost)y=asint and xsint-(1+cost)y+asint=0 is
1) x2-y2=a2 3) y2 = ax
2)x2+y2=a2 4) x2 = ay
Ans. Rearranging the above equations, we get
1-cost/sint=a-x/y and 1+cost/sint=a+x/y
multiplying the above equations ,we get x2+y2=a2
Ques. Lines ax+by+c=0, where 3a+2b+4c=0 & a,b,c all belong to the set of real numbers that are concurrent at the point?
1) (3,2) 3) (3,4)
2) (2,4) 4) (3/4, 1/2)
Ans. We know that,
3a+2b+4c =0
which equals (3/4)a+(1/2)b+c=0
So, the line passes through (3/4,1/2).
Ques. If the area of the triangle formed by the equation 8x2-6xy+y2=0 and the line 2x+3y=a is 7 then the value of a is ?
1) 14 3) 7
2) 28 4) 17
Ans. Equation of the sides of the given triangle are y=2x, y=4x and 2x+3y=a
So, vertices of the triangle are (a/8,a/4);(a/14,2a/7) and (0,0)
By determinant method, the area of triangle formed by these coordinates comes out to be a2/112 which is equal to 7
This gives the value of a as 28.
Ques. If the line x=k; k= 1,2,3,.....,n meet the line y=3x+4 at the points Ak(xk,yk), k= 1,2,3.....,n then the ordinate of the centre of the mean position of points Ak, k= 1,2,3,.....,n is
1) n+1/2 3) 3(n+1)/2
2) 3n+11/2 4) none of the above
Ans. We have yk=3k+4, the ordinate of intersection of x=k and y=3x+4 . So the ordinate of the mean position of the points Ak k= 1,2,3,.....,n is
(1/n){sum of all yk's} which comes out to be 3n+11/2
Ques. If the point (3,4) lies on the locus of the point of intersection of the lines xcost+ysint =a and xsint-ycost=b, the point (a,b) lies on the line 3x-4y=0 then |a+b|=?
1) 1 3) 7
2) 3 4) 12
Ans. Squaring and adding the given equations of the lines we get
x2+y2=a2+b2 as the locus of the point of intersection of these lines.
Since (3,4) lies on the locus we get
9+16=a2+b2
Also (a,b) lies on 3x-4y=0 so 3a-4b=0
Solving the two equation for a and b , we get |a+b|= 7
Ques. Equation of the circle with centre (-4,3) touching internally and containing the circle x2+y2=1is
1) x2+y2+8x-6y+9=0 3) x2+y2-8x+6y+9=0
2)x2+y2+8x-6y+11=0 4) x2+y2-8x+6y-11=0
Ans. Let the equation of the required circle be (x+4)2+(y-3)2=r2
If the above circle touches the circle x2+y2=1 internally, then the distance between the centres of the circles is equal to the difference of their radii
42+32=r-1
Which implies r=6
So the equation of the circle is x2+y2+8x-6y-11=0
Ques. If the normal chord at a point ‘t’ on the parabola y2=4axsubtends a right angle at the vertex, then the value of t is
1)4 3) 3
2)1 4) 2
Ans. Equation of the normal at ‘t’ to the parabola y2=4ax is y= -tx+2at+at3
The joint equation of the lines joining the vertex to the points of the intersection of parabola and the normal is
y2=4ax[y+tx/2at+t3]
4tx2-(2t+t3)y2+4xy=0
Since, these lines are at right angles so coefficient of x2+y2=0
So, t comes out to be 2.
Vectors and 3D geometry
- This topic closely resembles coordinate geometry. All the concepts from the basic coordinate geometry are exactly mirrored here except for the fact that, in 3D geometry there is an additional room for a third coordinate which is the ‘z coordinate’.
- The topic rather tests your ability to actually see and imagine the problem, rather a mental picture of the problem is necessary.
- It covers some of the 3D structures such as tetrahedron, parallelepiped and a sphere too.
- Unlike in coordinate geometry, where all of the calculations are based on two coordinates viz x and y, a new concept where unit vectors namely i, j and k are required to represent a coordinate in 3D geometry.
- JEE Main has recently scaled up the number of questions related to this topic, but scoring marks here is easy. Although, at first it takes some time to get acquainted with the third coordinate, once the concept is clear, solving 3D geometry questions becomes a routine task.
- In JEE Main expected number of questions from 3D geometry and vectors hovers somewhere around roughly 4 to 5 questions. (According to the previous years’ trends). Looking at the previous years’ JEE Main paper, on an average a total 16 questions were asked from 12th syllabus, out of which 4 were from this topic (25% weightage in 12th syllabus and 13.3% weightage overall).
- Topics covered under these units in JEE syllabus are – 3D geometry: Coordinates of a point in space, distance between two points, section formula, direction ratios and direction cosines, angle between two intersecting lines, Skew lines, the shortest distance between them and its equation, Equations of a line and a plane in different forms, intersection of a line and a plane, coplanar lines,tetrahedron, parallelepiped,sphere; Vectors and vector algebra – Vector addition, the component of vectors in two dimensional and three-dimensional space, scalar and vector products, scalar and vector triple product In vectors and vector algebra JEE asks questions based on triple products mostly
- In JEE Advanced syllabus, the expected number of questions from these topics vary a lot from year to year. JEE Advanced mostly asks around 40% to 50% questions from the 12th syllabus, of which you can expect around 15 to 20 percent questions from these topics which amounts to approximately 4 questions.
Best books for preparing Vectors and 3D Geometry are:
- For this topic solving previous year JEE Main question papers are strongly recommended. They have all types of questions of 3-D geometry and are enough to test acquired knowledge.
- Apart from this, Vector and 3D geometry for JEE Main and Advanced by Amit M Agarwal is a great book owing to a variety of questions and solved examples.
Some Frequently Asked Questions related to the above topics are:
Ques. The angle between a diagonal of a cube and one of its edges is,
1) cos-1(1/3) 3) /3
2) /4 4) /6
Ans. Let a= a1i, b=a1j ,c=a1k.
Then the vector d= a1(i+j+k) is a diagonal of the cube. The angle between one of the edges a, b or c and the diagonal d is given by,
cos=a.d/|a||d| which comes out to be cos-1(1/3).
Ques. Volume of the tetrahedron with vertices P(-1,2,0) ; Q(2,1,-3) ; R(1,0,1) and S (3,-2,3) is
1) 1/3 3) 1/4
2) 2/3 4) ¾
Ans. Volume of the tetrahedron is given by a scalar triple product,
Volume of tetrahedron= ⅙|PQ.(PR x PS)|
Here PQ, PR and PS are three vectors made from the above provided coordinates.
So, the volume of the tetrahedron comes out to be ⅔ after solving the triple product.
Ques. If A,B,C and D are four points in space and |ABxCD+BCxAD+CAxBD|=(area of triangle ABC). Then the value of is-
1) 1 3) 3
2) 2 4) 4
Ans. Let D be the origin of reference and DA=a, DB= b, DC= c
So, |ABxCD+BCxAD+CAxBD|= |(b-a) x (-c) +(c-b) x (-a) + (a-c) x (-b)|
= 2|axb+bxc+cxa|
=2(2 area of ABC)
Hence equals 4
Ques. A unit tangent vector at t=2 on the curve x=t2+2,y=4t3-5,z=2t2-6t is?
Ans. The position vector of any point at t is r=(t2+2)i+(4t3-5)j+(2t2-6t) k
dr/dt=2ti+12t2j+(4t-6)k
At t=2, the above comes out to be 4i+48j+2k, and the unit vector comes out to be (1/580) 2i+24j+k
Ques. Let N be the foot of the perpendicular of length p from the origin to a plane and l,m,n be the direction cosines of ON, the equation of the plane is
1) px+my+nz=l 3) lx+my+pz=n
2) lx+py+nz =m 4) lx+my+nz=p
Ans. The coordinates of N are (pl,pm,pn) and let P(x,y,z) be any point on the plane. The direction cosines of PN are proportional to x-pl, y-pm and z-pm. Since ON is perpendicular to the plane, it is perp. To PN
Hence, l(x-pl)+m(y-pm)+n(z-pm)=0
lx+my+nz=p(l2+m2+n2)=p, which is the locus of P and is the require equation of the plane.
Ques. The image of the point (-1,3,4) in the plane x-2y = 0 is
1) 8,4,4 3) 15,11,4
2) 9/5, -13/5,4 4) 4,4,1
Ans. Required image of the line lies on the line through A(-1,3,4) and perpendicular to x-2y=0 that is on the line
x+1/1 = y-3/-2 = z-4/ 0 =t (say)
So, the coordinates of the image is (t-1,-2t+3,4)
This point also lies on the plane, so t comes out to be 14/5
So, the required image is (9/5, -13/5,4).
Ques. If (2,3,5) is one end of the diameter of the spherex2+y2+z2-6x-12y-2z+20=0 then the coordinates of the other end are
1) 4,9,-3 3) 4,3,3
2) 4,-3,3 4) 4,3,5
Ans. Let the other end of the diameter be (a,b,c) , then the equation of the sphere is (x-2)(x-a)+(y-3)(y-b)+(z-5)(z-c)=0
Which equals x2+y2+z2-(2+a)x-(3+b)y-(5+c)z+2a+3b+5c=0
Comparing the above equations of the sphere with the equation given and comparing the corresponding terms we get,
The required coordinates as (4,9,-3).
Statistics and Probability
- Probability, a popular topic in IIT JEE Mathematics syllabus and a nightmare for many! Sometimes this chapter even challenges the brightest student’s patience and aptitude. Probability is a bit of a different topic than others such as Calculus, Trigonometry or Coordinate Geometry since Probability focuses more on the logical understanding and imagination of an individual. Rather than memory, calculation, solving equations etc.
- Statistics is not included in JEE Advanced, but it’s included in the JEE Main Syllabus and it’s very easy to score 100% in questions from Statistics. Moreover Statistics, unlike other topics, doesn’t need more time to master. Variance and Standard Deviations are usually asked, so formulas of Variance and Standard Deviation must be on tips.
- Probability finds a good place in JEE Advanced as well as JEE Main Papers and questions from the same are seen every year. Generally, JEE Advanced has 2-3 Questions from Probability which comprises 6-7% of Paper. While JEE Main comprises 9-10% of Questions from Statistics and Probability.
- In recent years Questions from Probability generally come in the form of Paragraphs that usually includes Bayes Theorem, Total Probability, and Binomial Distribution, hence Special Attention needs to be given to these topics.
- The key to mastering probability lies not just in rigorous and repetitive practice but it is also imperative to get the fundamentals strong. Before beginning with Probability, the knowledge of Permutation and Combinations & Sets is essential.
- Concepts such as Principle of Counting, Combinations, Group Formation, Selection among r elements, Venn Diagram are widely used while dealing with Probability Questions. Hence before beginning with Probability make sure to clear your concepts of PnC otherwise you’ll get bowled out.
Best Reference Books for preparing JEE Main Probability and Statistics:
- NCERT and NCERT Exemplar
- Mathematics for JEE Advanced Algebra Cengage Algebra by G..Tewani
- Skills in Mathematics for JEE Mains and Advanced Algebra by S.K Goyal
- Problem Plus in Mathematics by A Das Gupta
- Previous Year JEE Question Papers
Some Important Questions which you must have a look at:
Example 1: Four Persons independently solve a certain problem correctly with probabilities ½, ¾, ¼ and 1/8. Then the probability that the problem is solved correctly by at least one of them. [JEE Advanced 2013]
Solution: P(Problem solved by at least one of them)=1-P(Problem solved none of them)
=1-½*¼*¾*7/8
=1-21/256
=235/256
Example 2: An unbiased coin is tossed. If the result is head, a pair of unbiased dice is rolled, and the number obtained by adding the number on the two faces are noted. If the result is a tail, a card from a well-shuffled pack of 11 cards numbered 2, 3, 4.... .., 12 is picked & the number on the card is noted. What is the probability that the number noted is 7 or 8?
Solution: Let us define the events:
A : head appears.
B : Tail appears
C : 7 or 8 is noted.
We have to find the probability of C i.e. P (C)
P(C) = P(A) P (C/A) + P(B) P(C/B)
Now we calculate each of the constituents one by one P(A) = probability of appearing head=½
P(C/A) = Probability that event C takes place i.e. 7 or 8 being noted when head has already appeared. (If something has already happened then it becomes certain, i.e. now it is certain that head has appeared we have to certainly roll a pair of unbiased dice).
= 11/36 (since (6, 1) (1, 6) (5, 2) (2, 5) (3, 4) (4, 3) (6, 2) (2, 6) (3, 5) (5, 3) (4, 4) i.e. 11 favorable cases and of course 6 × 6 = 36 total number of cases)
Similarly, P(B) = 1/2
P(B/C) = 2/11 (Two favorable cases (7 and 8) and 11 total number of cases).
Hence, P(C) = ½ × 11/36 + ½ × 2/11 = 193/792 (Ans.)
Example 3: Sixteen players P1, P2, ….. P16 play in a tournament. They are divided into eight pairs at random. From each pair, a winner is decided on the basis of a game played between the two players of the pair. Assuming that all the players are of equal strength, the probability that exactly one of the players P1 and P2 is among the eight winners is
(a) 4/15
(b) 5/9
(c) 3/8
(d) 8/15
Solution: Let E1 and E2 denote the event that P1 and P2 are paired or not paired together. Let A denote the event that one of the two players P1 and P2 is amongst the winners.
Since, P1 can be paired with any of the remaining 15 players, so P(E1) = 1/15
and P(E2) = 1 – P(E1) = 1 – 1/15 = 14/15
In case E1 occurs, it is certain that one of P1 and P2 will be among the winners. In case E2 occurs, the probability that exactly one of P1 and P2 is among the winners is
P[(P1 ∩ P2C) ∪ (P1C ∩ P2)] = P(P1 ∩ P2C) + P(P1C ∩ P2)
= P(P1) P(P2C) + P(P1C) P(P2)
= ½ (1 - 1/2) + (1 - 1/2)1/2
= ¼ + ¼
= ½
i.e. P(A/E1) = 1 and P(A/E2) = ½
By the total probability rule,
P(A) = P(E1). P(A/E1) + P(E2) P(A/E2)
= 1/15 (1) + 14/15(1/2)
= 8/15
Example 4: In a test an examinee either guesses or copies or knows that answer to a multiple choice question which has 4 choices. The probability that he makes a guess is 1/3 and the probability that he copies is 1/6. The probability that his answer is correct, given the copied it, is 1/8. Find the probability that he knew the answer to the question, given that he answered it correctly.
Solution:
P(g) = probability of guessing = 1/3
P(c) = probability of copying = 1/6
P(k) = probability of knowing = 1 - 1/3 - 1/6 = ½
(Since the three-event g, c and k are mutually exclusive and exhaustive)
P(w) = probability that answer is correct
P(k/w) = (P(w/k).P(k))/(P(w/c)P(c) + P(w/k)P(k) + P(w/g)P (g)) (using Baye's theorem)
= (1×1/2)/((1/8,1/6) + (1×1/2) + (1/4×1/3) )
= 24/29(Ans.)
Example 5: A speaks truth 3 out of 4 times. He reported that Mohan Bagan has won the match. Find the probability that his report was correct.
Solution:
Method 1:
Let T : A speaks the truth
B : Mohan Bagan won the match
Given, P(T) = 3/4
.·. P(TC) = 1 - 1/3 = 1/4
A match can be won, drawn or loosen
.·. P(B/T) = 1/3 P(B/TC) = 2/3.
Using Baye's theorem we get
P(T/B) = (P(T).P(B/T))/(P(T).P(B/T) + P(TC)P(B/TC))
= 3/4×1/3)/(3/4×1/3 + 1/4×2/3) = (1/4)/(5/12)
=3/5
Method 2:
Let, T : The man speaks truth
A : Mohan Bagan won the match
B : He reported that Mohan Bagan has won.
P(A) = 1/3(the match may also end in a draw)
P(T) = ¾
P(B) = P(A) P(T) + P(AC) P(TC)
= 1/3×3/4 + 2/3×1/4
= ¼ + 1/6
= (3+2)/12 = 5/12
P(T/B) = (P(B/T).P(T))/(P(B)) = (1/3×3/4)/(5/12)
= 3/5 (Ans.)
Question-Solving Tips for JEE Main 2023 Mathematics
- Solve Everyday: Mathematics is one subject that you can’t miss out on practicing on even for a single day. Candidates should make sure that they practice 20 -25 questions of mathematics every day.
- By the Clock: Candidates must make sure that they are going by the clock when they solve mathematical problems. If you fail to complete the questions in a given time limit, try to set a time limit for every question.
- Classify Your Problems: When you solve questions, you can label them as ‘E, M and D’. E for the questions that you found easy and don’t need to be looked at during the time of revision. M for the questions which you found somewhat challenging and you need to look at them once during the time of revision. D for the questions you found very difficult and you must solve them in order to keep the concepts fresh.
- Back your approach with a logical sequence: It is best to back your approach while solving the problem with logic, avoid guesswork at every step as much as possible. An accurate logical sequence will bring conceptual clarity.
- Day wise Targets: Mathematics syllabus is vast and dynamic, don’t panic by looking at how much is still left to cover. Candidates can set day-wise targets beforehand and make sure they stick to it.
- End your day with analyzing: Just practicing randomly won’t do any good, You must keep a track of your mistakes and weak spots. Analyze whatever you’ve studied during the day and overcome your weaknesses.
Best Reference Books to Cover JEE Main 2023 Mathematics Syllabus
| Books and Authors | Best Suitable For |
|---|---|
| Maths for Class XI and XII by R.D. Sharma | Understanding of Basics |
| Maths for Class XI and XII by R.S. Agarwal | Understanding of Basics |
| Plane Trigonometry by S L Loney | Trigonometry |
| The Elements Of Coordinate Geometry by S L Loney | Coordinate Geometry |
| Algebra by Dr S K Goyal Arihant Publications | Algebra |
| Geometry by Dr Gorakh Prasad | Geometry |
| Play with Graphs by Amit M Agarwal (Arihant Publications) | Practicing Problems |
| Differential Calculus by Amit M Agarwal (Arihant Publications) | Calculus |
| Integral Calculus by Amit M Agarwal (Arihant Publications) | Calculus |
Candidates must not forget to cover NCERT of Class XI and XII thoroughly as test takers often have reviewed it as the best source of preparation for handling JEE Main Question Papers.
Must Read Interviews of JEE Main Toppers (NTA Score 100)
- JEE Main Topper Rongala Arun Siddardha
- JEE Main Topper Landa Jitendra
- JEE Main Topper Parth Dwivedi
- JEE Main Topper Chagari Koushal Kumar Reddy
Video Lectures by IIT Faculty for JEE Main Mathematics Preparation
Mathematics online video lectures by IIT faculty can prove to be highly beneficial for the candidates. The links for the video lectures can be accessed from the NTA website. The steps to access the video lectures are as follows:
- Step 1: Visit the official website of NTA i.e. nta.ac.in.
- Step 2: Click on the “CONTENT BASED LECTURES - FOR JEE MAIN AND NEET-UG BY IIT PROFESSORS / SUBJECT EXPERTS” tab.
- Step 3: You will be redirected to the page containing the name of different subjects.
- Step 4: Choose the video lecture by clicking on the subject.
Direct Link to JEE Main Mathematics Video Lectures
Difficulty Level Analysis for JEE Main Mathematics
The level of difficulty of the JEE Main Mathematics section varies in different sessions. It often comes across as the most challenging and time consuming section to the test takers. For the understanding of candidates, a basic distribution of number of questions in terms of difficulty level of B.E/ B. Tech paper of JEE Main 2020 January session is tabulated below:
| Sections | Tough | Medium | Easy |
|---|---|---|---|
| Chemistry | 4 | 11 | 10 |
| Mathematics | 2 | 10 | 13 |
| Physics | 2 | 7 | 16 |
| Sections | Tough | Medium | Easy |
|---|---|---|---|
| Chemistry | 4 | 11 | 10 |
| Mathematics | 2 | 10 | 13 |
| Physics | 2 | 7 | 16 |
Mathematics section for the January B.E/ B.Tech paper can be defined as of a medium difficulty level overall but candidates must keep in mind that level of difficulty is a subjective concern and different candidates can have a different perspective on the question paper, All the Best!
JEE Main Mathematics Syllabus FAQs
Ques. Is the JEE Main mathematics syllabus different for BE/BTech, BArch and BPlanning?
Ans. No, the JEE Main 2023 mathematics syllabus is the same for all three papers.
Ques. Are definite integrals part of the JEE Main Mathematics syllabus?
Ans. Yes, definite integrals are a part of the syllabus, under the integral calculus section.
Ques. What topic has the highest weightage in the JEE Main mathematics syllabus?
Ans. According to previous years trends, both coordinate geometry and differential calculus have the highest weightage with each carrying 17% of the total marks of the paper.
Ques. What is the most scoring part of the JEE Main mathematics syllabus?
Ans. The Coordinate geometry section is one of the most scoring parts of the JEE Main mathematics syllabus as it is mostly formula based.
Ques. What is the trickiest part of the JEE Main mathematics syllabus?
Ans. The Probability section of the JEE Main mathematics syllabus can be quite challenging as it focuses on testing the student’s logical understanding and imagination.
Ques. How do I tackle the large JEE Main mathematics syllabus properly?
Ans. The systematic approach to prepare for JEE Main mathematics syllabus is to divide it up into smaller sections and practice a few of those sections every day while solving sample papers to find your problematic areas.
*The article might have information for the previous academic years, which will be updated soon subject to the notification issued by the University/College.






Comments